
Mechanics is a branch of Physics that deals with the study of motion and forces. Class 11 Mechanics is the grammar of Physics and one major part of the NCERT syllabus. We offer an exhaustive series of class 11 Mechanics video lectures on all chapters.
Students of class 11 and 12 under CBSE, ISC, or any other provincial Board, if you aspire to succeed in competitive examinations like IIT-JEE or NEET, you will learn everything that you need to learn from our videos. The lectures lucidly explain the basic theories in detail, and solve a large number of problems – both simple and advanced – in a graded manner to hone your problem-solving skill. Our lectures will be very useful to any student preparing for any Physics-based Board or competitive exams anywhere in the world.
NCERT assigns 40 marks to Mechanics-based questions out of 70 marks in the Class XI CBSE exam paper (theory). In recent IIT-JEE Main papers, questions on Mechanics get the maximum weightage of about 29-30%. In recent NEET papers, Mechanics gets a weightage of about 19-20%.
In class 11 Mechanics video lectures we cover the following topics:
Unit I : Physical World and Measurement
In class XI physics course, the topic of "Physical Quantities and Their Measurements" is a useful introduction. A physical quantity is measured in terms of a small part of it. The small part is conventionally adopted as a unit of measurement of the quantity. It is helpful to first establish the units of a few quantities which are called base quantities or fundamental quantities. The corresponding units are called base units or fundamental units. The units of the remaining physical quantities are expressed in terms of these base units. These quantities are called derived quantities, and their units are called derived units. The set of base and derived units is called a system of units.
In 1960, an international committee called CGPM adopted the International System of Units or SI which has been supplemented and refined ever since. The group of SI units consists of seven base units and two supplementary units.
The concept of order of magnitude is often very helpful to compare the magnitudes of two or more physical quantities of the same type. At first the absolute value of a given quantity is written in the form
x × 10y where 1≤ x< 10 and y is a positive or negative integer. Next, the number is rounded off according to specific guidelines. The power of 10 in the final representation is called the order of magnitude of the quantity. For instance, the mass of the earth is
about 5.98 × 1024 kg and the mass of an electron is about 9.11 × 10-31 kg.
By the above rules, the mass of the earth is of the order of 1025kg and the mass of an electron is of
the order of 10-30 kg .
Therefore, the mass of the earth is 25 - (-30) = 55 orders of magnitude larger than the mass of an electron.
The word dimension in physics means the physical nature of the quantity.
The dimensions of a quantity should not be confused with its units. To illustrate the point,
the distance between two places can be measured in different units such as metre, centimetre, foot etc. But the dimension of
distance is always the same, which is "length". The usual practice is to use square brackets, [ ], to denote the dimensions of a
physical quantity. Thus, the dimension of distance is [L] , the dimension of mass is [M],
the dimensions of velocity and force are [LT-1] and [MLT-2] respectively.
Any equation that relate several physical quantities must be homogenous from the dimensional point of view. If an equation is of the form A = B + C, then A, B and C must represent the same physical quantity with the same dimensions. This property is known as the principle of homogeneity of dimensions. To illustrate the point, let us take an important equation from Kinematics:
x - x0 = ut + ½ at2
Here (x-x0) is the distance travelled by a particle along a straight line in time t, u is the initial velocity of
the particle and a is the constant acceleration with which the particle is moving.
The dimension of (x - x0) is [L], the dimension of ut is [LT-1][T] = [L],
and the dimension of at2 is [LT-2][T2] = [L]. Therefore,
the dimensional form of the equation can be written as:
[L] = [L] + [L]
which demonstrates that the equation is dimensionally homogenous.
The method of dimensional analysis has many uses:
Each one of them will be discussed with examples in due course.
We can never measure a physical quantity with absolute precision. The degree of accuracy of any measurement depends on the smallest scale division of the measuring instrument. This is known as the least count of the instrument. The least count of an ordinary metre scale is 0.1 cm or 1 mm. When we use it to measure the length of a certain steel rod, suppose the reading falls somewhere between 24.6 cm and 24.7 cm marks. So we divide the space between the two marks mentally in 10 equal parts and guess how many of these parts are the from the 24.6 cm mark to the end of the rod. Let this number be 3. Accordingly, the length of the rod is taken as 24.63 cm. So we see here that the first three digits counted from the left, i.e. 2, 4 and 6 are certain but the fourth digit 3 is uncertain. The significant figures of any measurement are the digits which are reasonably reliable. By convention, they consist of all the certain digits and only one uncertain digit. Thus, a metre scale can read up to four significant figures (2, 4, 6 and 3 in our example).
Certain points should be kept in mind while counting significant figures in a number. Certain rules are to be followed in counting significant figures in the answer of algebraic operations such as multiplication, division, addition and subtraction. They will be discussed in due course.
Any experiment involves a series of measurements. Several errors may occur during these measurements. The errors in measurement can be classified into two basic types: systematic error and random error.
Often we wish to find the value of a quantity Z that involves the measurement of two other quantities X and Y.
The combined error in the value of Z depends not only on the errors in the values of X and Y, but also on the nature of
dependence of Z on X and Y. There are specific formulas which we can develop to calculate the
combination of errors for various cases such as
Z = X + Y, Z = X - Y, Z = XY, Z = X / Y, Z = Xn,
or a more general type U = k Xa Yb / Zc.
The physical quantities which we encounter in our daily lives may be broadly divided into two groups: scalars and vectors. A quantity which is completely specified by a number with an appropriate unit is called a scalar. It has only magnitude but no direction. Examples are mass, distance, time, speed, work, temperature, volume etc. A quantity which is completely specified by a number with an appropriate unit and a direction is called a vector. Thus, it has both magnitude and direction. Some examples of vectors are displacement, velocity, acceleration, force, momentum, torque, gravitational field etc.
Vector quantities follow the rules of vector algebra, which are different from the rules of arithmetic. This is an important criterion for checking whether a quantity is vector or not, as we shall see when we study Rotational Mechanics.
The graphical representation of a vector is a straight line with an arrowhead on one tip. The length of the line segment represents the magnitude of the vector on a convenient scale; the arrowhead gives the direction of the vector.
Three or more vectors which are in the same plane or are parallel to the same plane are called coplanar vectors. Equal vectors are those which have the same magnitude, same or parallel lines of support, and the same sense. The negative of a vector is the one whose magnitude is equal to that of the given vector, but the direction is opposite. A zero vector or null vector is the one which has zero magnitude and no specific direction. The position vector of a point represents the position of the point with respect to the origin of any coordinate system. The unit vector is obtained by dividing a vector by its magnitude. The magnitude of a unit vector is 1 (no unit), and its direction is the same as that of the original vector. As an example, if a force vector is 5 N directed southwards, the corresponding unit vector will be 1 due south.
The rules of addition of vectors are more complicated than those of arithmetic addition. The addition gives a resultant vector which produces the same effect as the combining vectors produce together. Two vectors can be added, both graphically and analytically, by the triangle law of vector addition or parallelogram law of vector addition. Several vectors can be added graphically by the polygon law of vector addition. For a more accurate addition of several vectors, analytically, we make use of addition of vectors by resolution method.
The subtraction of vectors follows similar approach as addition of vectors, with an important difference. The subtraction of one vector from another is defined as the addition of the negative of the vector to be subtracted. If P and Q are two vectors with the same unit then:
P - Q = P + (- Q)
The resolution of a vector is the process of determining a set of vectors which produce together the same effect as the original vector does alone. Each vector in the set is known as a component vector of the original vector. Thus, the process of resolution is opposite to the process of vector addition. Among many possibilities of resolution, the most useful ones are the resolution of a vector on a plane along two mutually perpendicular x- and y-axes AND the resolution of a vector in space along three mutually perpendicular x-, y- and z-axes.
Like addition and subtraction, the multiplication of vectors follows a set of rules different from those of arithmetic multiplication. The vectors to be multiplied need not have the same unit. There are two types of product known as the scalar product or dot product AND vector product or cross product.
The scalar product of two vectors is a scalar quantity equal to the product of the magnitudes of the given vectors and the cosine of the angle between them. The physical quantity "work" is the scalar product of force vector and displacement vector.
The vector product of two vectors is a vector quantity whose magnitude is equal to the product of their individual magnitudes and the sine of the smaller angle between them. The direction of the vector product is perpendicular to the plane containing the given vectors and its sense is determined by the right-hand corkscrew rule or the right-hand thumb rule, discussed in due course. The physical quantity "torque"is the vector product of displacement vector and force vector.
Historically, the term mechanics was first used by Sir Isaac Newton to mean the science of machines and the art of making them. Today we use the word to mean the branch of science that describes and predicts the conditions of rest or motion under the effect of a set of forces. Mechanics can be divided into two parts: dynamics and statics. Dynamics is the study of motion of a body under one or more forces. Statics is the study of the condition of rest of a body under a number of forces. Dynamics is further divided into kinematics and kinetics. Kinematics is that part of dynamics which deals with motion ignoring the forces that cause it or the properties of the body in motion. Presently, we shall deal with kinematics here. Kinetics is that part which relates the motion of a body to its mass and the causal force(s). Newton's laws of motion , which are a fundamental part of kinetics, will be dealt next.
A body is a portion of matter which extends over a certain region of space and has a definite shape and size. But the study of motion of a body can be made simpler by ignoring its size and shape, and, assuming that the whole mass of the body is concentrated at a point occupying a definite position in space. In that event, we refer to the body as a particle. The particulate view of body is valid in many practical situations. The size of the earth and the sun are huge in absolute terms, but both are much smaller than the distance between them. Therefore, when we study the motion of the earth round the sun, we can treat both of them as particles!
When we observe a nearby object on the earth's surface, we intuitively determine whether it is at rest or in motion with respect to the ground. But considering the fact that the ground itself is in motion (earth rotates about its own axis and, simultaneously, revolves round the sun), the difference between rest and motion is not as clear-cut as one may think. The early scientists who searched for an absolutely fixed body met with little success. It was Albert Einstein who finally suggested that there is nothing called absolute rest or absolute motion. Rest and motion are purely relative concepts, and the significant thing is whether the body under investigation is at rest or in motion with respect to its local surroundings. These local surroundings are known as the frame of reference.
The frame of reference is usually pictured in terms of a three-dimensional rectangular coordinate system, often called cartesian coordinate system, consisting of three mutually perpendicular axes intersecting at a point. The axes are designated as x-, y- and z-axes; the point of intersection, marked by the letter O, is known as the origin of the system.
The motion of rigid bodies can be of two types: translational motion and rotational motion. When a rigid body performs translational motion, all particles constituting the body travel with the same velocity and acceleration at any given instant. Hence the paths followed by them have the same length and are parallel to each other. If these paths are straight lines, we say the body is in rectilinear motion (that is, motion in a straight line). Example: motion of a car along a flat, straight, narrow road. If the paths of the particles are curved, the body is said to be in curvilinear motion. An interesting example is the motion of a wooden horse on a merry-go-round.
When a rigid body performs rotational motion, the particles of the body located at different radial distances from the axis of rotation travel with different velocities and accelerations at any given instant. Hence the paths followed by them have different lengths. Example: motion of a fan blade or a spinning wheel.
The motions we see around us are often a combination of translation and rotation. The rolling motion of a sphere on the floor is one such example.
Presently, we shall study translational motion in one dimension and in two dimensions. The study of pure rotational motion and rolling motion will be done later within Mechanics.
Here are some terms associated with translational motion. Suppose a particle which is at position P1 at time t1 moves to a new position P2 at time t2 following a curved path P1P3P2 .
The length of the actual path gives the distance travelled by the particle. Distance is a scalar quantity without direction. The displacement, on the other hand, is given by the straight line P1P2 which joins the initial and final positions of the particle. Displacement is a vector quantity. The magnitude of displacement is the length of the straight line P1P2 , and its direction is from P1 to P2 . Both distance and displacement have the same dimension of length, [L], and have the same SI unit metre (m).
The speed of a moving particle is the distance travelled by it in unit time. Speed is a scalar quantity. The velocity of a particle is its displacement per unit time. Velocity is a vector quantity. Both speed and velocity have the same dimensions, [LT -1] , and have the same SI unit metre per second (m/s).
The acceleration of a particle is the rate of change in velocity with time. The change in velocity may be due to a change in its magnitude, direction or both. Like displacement and velocity, acceleration is a vector quantity. The dimensions of acceleration are [LT-2], and its SI unit is metre per second square (m/s2). Negative acceleration is known as deceleration or retardation.
Graphical analysis of rectilinear motion, as the name suggests, is the visualisation of a particle's straight-line motion with the help of a graph. Two commonly plotted graphs are position versus time graph and velocity versus time graph.
The simplest example of accelerated motion is rectilinear motion with constant acceleration. It is possible to develop a useful set of kinematic equations either by graphical method or by the method of calculus (integration). These equations involve quantities such as displacement, time, initial and final velocities, and acceleration. The vertical motion of a freely falling body under gravity is a case of rectilinear motion with constant acceleration. A more complicated case is rectilinear motion with variable acceleration. When a body moves back and forth about a point in simple harmonic motion (a special case of periodic motion), its acceleration varies as a function of its displacement. Problems of this type will also be dealt with in due course.
The kinematic equations developed for rectilinear motion can be suitably modified to analyse motion in a plane, that is, motion in two dimensions. If a particle is thrown obliquely in air near the earth's surface, it follows a curved path that lies in a vertical plane. The particle is called a projectile, and the path followed by it is called a trajectory. A bullet fired from a gun or a ball hit by a cricket bat is an example of a projectile. Assuming acceleration due to gravity, g, remains constant throughout and air resistance is negligible, projectile motion is an example of motion in a plane with constant acceleration.
Other examples of two-dimensional motion are circular motion and motion of a boat in a river.
The relative velocity of a body with respect to another body is defined as the rate of change of position of the first body as observed by the second body. It is given by the vector difference between their absolute velocities. Similarly, the relative acceleration of a body with respect to another body is defined as the rate of change of velocity of the first body as observed by the second body. This is given by the vector difference between their absolute accelerations.
Kinetics is the study of how the motion of a body is related to its mass and the force(s) acting on it. The force represents the interaction of a body with its environment. The mass of a body is a measure of its inertia, which is the tendency to resist acceleration under a force. Newton's laws of motion are fundamental to the study of kinetics.
Newton's three laws of motion were first published in his celebrated work
Mathematical Principles of Natural Philosophy in the year 1687. We shall touch upon each of them.
Newton's first law of motion: A body at rest continues to remain at rest and a body in motion continues in motion with a constant velocity unless it is compelled by a net external force to change that state.
The statement of the first law provides two important concepts:
Newton's first law is often called the law of inertia. Any frame of reference in which Newton's first law is valid is called an inertial frame of reference. Qualitatively, a force is defined as an external agent which, when applied to a body, changes its state of rest or of motion with a constant velocity.
Newton's second law of motion: The rate of change in momentum of a body is proportional to the net external force that acts on the body and it takes place in the direction in which the force acts. The statement of the second law provides two more concepts:
Momentum is given by the product of the mass and velocity of a particle. Since mass is a scalar and velocity is a vector, momentum is a vector quantity which has the same direction as velocity. The dimensions of momentum of are [MLT-1], and its SI unit is kilogram metre per second (kg-m/s).
Quantitatively, force is given by the product of mass and acceleration. Since mass is a scalar and acceleration is a vector, force is a vector quantity which has the same direction as acceleration. The mathematical statement of Newton's second law is:
F = ma
This very important equation is called equation of motion. The dimensions of force are [MLT-2], and its SI unit is newton (N).
Newton's third law of motion: To every action there is always an equal and opposite reaction. In all cases, the action and reaction forces act on different bodies.
Whenever a particle 1 exerts a force of action, F21, on another particle 2, the latter also exerts a force of reaction, F12, on the former. These two forces are equal in magnitude and opposite in direction. They act along the same line joining the two particles. Therefore, we can write:
F12 = -F21
The above relationship is mutually reciprocal. That is, either of the two forces can be thought to be the action force and the other, the reaction force. Another important aspect of the third law is, even though forces always occur in pairs, they always act on two different bodies and do not cancel each other. If they were to act on the same body, the net force on the body would be zero and it could never have an acceleration!
Newton's second and third laws can be applied to explain a variety of events around us. But the first and foremost step towards that is to learn how to draw free body diagrams. Every time you want to write the equations of motion of a body, it is advised that you isolate the body from its surroundings, identify all the forces acting on it and show them clearly in a separate diagram. No need to show the bodies which exert the forces. The forces of reaction, which are exerted by the body under investigation, do not appear in the diagram. A force diagram of this type is known as a free body diagram. A correct free body diagram usually means correct equations of motion and a straightforward solution to the problem.
Among the many applications of Newton's second and third laws, problems on pulleys and wedges are of particular interest. The type of motion in which the velocities or accelerations of two or more particles depend on each other is known as constrained motion. When we analyse a constrained motion by applying Newton's laws, we must take into account additional conditions imposed on such a motion. This conditions are known as constraints. We shall discuss constrained motion of two types of system: pulley-block system and wedge-block system.
Any frame of reference, which accelerates with respect to an inertial frame of reference, is called a non-inertial frame of reference. Newton's laws are not valid in such a frame of reference. The net force acting on a particle in a non-inertial frame is made up of two parts: a real force and a fictitious force. The fictitious force, which has no material source, is called pseudo force and given by - ma0 , where m is the mass of the particle and a0 is the acceleration vector of the non-inertial frame with respect to the inertial frame. The pseudo force(s) do not exist when the motion is observed from an inertial frame.
We often simplify a problem by assuming that the motion of bodies takes place on "frictionless" surfaces. But in real world, all motions happening around us are affected by the force of friction. Therefore, a realistic approach to any mechanical problem requires that we identify the frictional forces acting on the system and include them in the respective equations of motion. Suppose a block 1 is placed on top of another block 2.
As long as the blocks are at rest and there is no attempt to produce a relative motion between them, the contact forces acting in a pair between the blocks are purely normal forces and directed perpendicular to the surface separating the blocks. But if a driving force F is applied to block 1 in an attempt to drag it to the right along the surface of block 2, the contact forces between the blocks act at an oblique angle to the surface of separation.
The components of contact forces perpendicular to the surface are the normal forces N and - N. The components of contact forces parallel to the surface are the frictional forces f and - f . By Newton's third law, these frictional forces always act in an action-reaction pair. Their directions are such that they resist any real or impending relative motion between the two blocks. In our case, since the driving force F is applied to the right, the frictional force on block 1 acts to the left and the frictional force on block 2 acts to the right.
The resistance to the relative motion between two solid bodies is known as solid friction, or simply friction. The resistance to the relative motion between a solid body and a fluid is known as fluid friction, or more commonly as drag force. The relative motion between two solid bodies can be produced in two different ways.
They can either slide over one another or they can roll over one another. Accordingly, solid friction can be classified into two types: sliding friction and rolling friction. And again, the friction that exists when the bodies are about to slide over one another is not the same as the friction that exists when the bodies are actually sliding over one another. The first type is called static friction, and the second type is called kinetic friction.
Consider a block of mass m resting on a rough, horizontal surface. A driving force F is applied rightwards to the block. As the magnitude of F is increased slowly, the force of static friction fs acting leftwards on the block also increases equally in magnitude to keep the block at rest. When the block is just about to move to the right, the force of static friction attains its maximum value which is called the force of limiting friction.
According to the laws of static friction, force of limiting friction is directly proportional to the magnitude of the normal force between the two surfaces. In other words, the ratio of force of limiting friction and normal force is a constant known as coefficient of static friction. Once the block begins to move, the force of kinetic friction fk comes into play, which is usually smaller than the force of limiting friction. By the laws of kinetic friction, force of kinetic friction is also directly proportional to the magnitude of normal force. The corresponding ratio of force of kinetic friction to normal force is a constant called coefficient of kinetic friction. Generally, the first coefficient is greater than the second for a given pair of surfaces.
It will be useful to introduce few more terms. Suppose a block rests on a rough plane inclined at a small angle to the horizontal. The angle of inclination is gradually increased until, at a certain point, the block is just about to slide down the incline. The corresponding angle of inclination of the plane is known as angle of repose.
The angle of friction between a given pair of surfaces is the angle between the total contact force and the normal force at the instant when one surface is just about to slide over another.
If the driving force acting on a block is slowly rotated through 360° keeping it parallel to the surface of the floor, the total contact force acting on the block remains within an imaginary inverted right circular cone, as long as the block is at rest. This cone is known as cone of friction.
The study of circular motion is not only important in itself, but also an essential pre-condition for the study of rotational motion later under Mechanics. We start with the definitions of the angular quantities in circular motion.
Suppose a particle is moving in a circle in the counterclockwise direction. The centre of the circle coincides with the origin O of the coordinate system Oxy. The initial position of the particle is P1 at time t1. The final position of the particle is P2 at a later time t2 . The angular displacement of the particle is equal to the angle swept by its position vector as it moves from OP1 to OP2 . Angular displacement is a pure number without dimensions. However, it is customary to express it in an artificial supplementary SI unit called radian (rad).
The angular velocity of the particle is its angular displacement per unit time. In uniform circular motion, the angular velocity has the same value at every instant. In nonuniform circular motion, the angular velocity keeps changing from one instant to another. The dimension of angular velocity is [T-1] ; its unit is radian per second (rad/s) or simply per second ( /s) since radian is a dimensionless unit. The time taken by a particle to complete one revolution is known as its time period. The number of revolutions completed by the particle per second is called its frequency.
In the case of nonuniform circular motion, the angular acceleration of a particle is the rate of change in angular velocity with time.The dimension of angular acceleration is [T-2] ; its unit is radian per second square (rad /s2), or just per second square ( /s2).
It is useful to note the relations between linear and angular quantities. A particle, moving in a circle of radius r, changes its position from P1 at time t1 to P2 at time t2 . The distance travelled by the particle is the length of the arc P1P2 . This distance is equal to the product of its angular displacement and radius of the circle. Similarly, the linear speed of a particle moving in a circle at any instant is the product of its angular speed and circle's radius.
The linear acceleration of a particle in circular motion has two components: tangential and radial. The tangential acceleration at any instant is the product of its angular acceleration and circle's radius. The radial acceleration, or centripetal acceleration as it is more commonly called, is the product of angular speed squared and circle radius.
Circular motion of a particle with constant angular acceleration is analogous to motion in a straight line with constant linear acceleration. Therefore, it is possible to develop a set of kinematic equations of circular motion in the same way as we did it for rectilinear motion. These equations involve quantities such as angular displacement, initial and final angular velocities, and angular acceleration.
When a particle performs uniform circular motion, its angular acceleration is zero, which means its tangential acceleration is also zero. The only acceleration present is the centripetal acceleration directed radially towards the centre of the circle. According to Newton's second law, the particle experiences a net external force – directed radially inwards – of magnitude given by the product of the mass of the particle and its centripetal acceleration. This force is known as centripetal force. An example of centripetal force is the gravitational force exerted by the sun on the earth which makes the latter revolve round the sun. In a rotating non-inertial frame of reference, a particle experiences a pseudo force directed radially outwards. This pseudo force is known as centrifugal force.
When a particle performs nonuniform circular motion, its tangential acceleration and centripetal acceleration are both non-zero quantities. Therefore, by Newton's second law, the net external force acting on the particle is the resultant of a tangential force and a centripetal force. A sphere moving in a vertical circle at the end of a light string constitutes a case of nonuniform circular motion.
The work done by a force on a particle is defined as the product of the component of the force in the direction of the displacement and the magnitude of the displacement. Suppose a constant force F acts on a block to produce a displacement s in it. The angle contained between the vectors F and s is θ . Then the work done by the force is:
W = Fs cos θ
Work is the scalar product of force and displacement vectors, which means work itself is a scalar and has no direction. If the angle between F and s is an acute angle (< 90°), work is positive. If the angle between F and s is an obtuse angle (> 90°), work is negative. If the angle between F and s is 90° , work done is zero. When a block moves on a horizontal floor, the force of gravity exerted by the earth and the normal force exerted by the floor do not do any work on the block because these forces act at right angles to the motion of the block. The dimensions of work are [ML2T-2], and its SI unit is joule (J).
To calculate the work done by a varying force, we need a more rigorous, calculus-based approach. Let us consider the case of a block attached to a spring. Suppose one end of a spring is attached to a fixed wall and the other end is attached to a block resting on a horizontal, frictionless floor. Initially, the spring is at its natural length. Now if an external force is applied to the block to move it slowly without acceleration further away from the wall, the spring stretches and exerts a variable restoring force opposite to the block's motion. The magnitude of this spring force is directly proportional to the displacement of the block.
The constant of proportionality is known as the force constant of the spring. If the spring is stretched to take the block from initial position x = 0 to final position x = x, it can be shown by integration that the work done by the external force is:
Wext= 1/2 kx2
The corresponding work done by the spring force is:
Wspr= -1/2 kx2
Here k is the force constant of the spring. Notice that the external force does positive work because it has the same direction as the block's displacement. The spring force does negative work (of the same absolute value) because it is directed opposite to the block's displacement.
The energy of a body can be loosely defined as its capacity of doing work, measured by the total amount of work the body can do. Energy can be of different types, e.g. mechanical, thermal, light, electrical, magnetic, sound, chemical and nuclear. In classical mechanics, we shall mostly deal with mechanical energy which is further classified into two types: kinetic energy associated with the motion of a body, and potential energy associated with the configuration of a system such as gravitational, elastic and electrical potential energy. All types of energy have the same dimensions and units as those of work.
The kinetic energy K of a particle of mass m moving with speed v is defined as:
K= 1/2 MV2
This is a scalar quantity which is always positive. According to the work-energy theorem, the net work done on a particle is equal to the change in its kinetic energy. In mathematical terms:
Wnet = 1/2 mv22 - 1/2 mv12 = K2 - K1 = ΔK
where v1 and v2 are the initial and final speeds, K1 and K2 are the initial and final kinetic energies, Δ K is the change in kinetic energy of the particle.
The power supplied by a force is defined as the rate at which the force does work. It is the scalar product of force and velocity vectors. That is:
P = F.v = Fv cos θ
where θ is the angle contained between F and v. The dimensions of power are [ML2T-3], and its SI unit is watt (W).
The potential energy of a system is the energy possessed by it by virtue of its configuration. It is measured by the work the system can do if it returns from its present configuration to some standard configuration usually associated with zero potential energy.
Forces in nature can be divided into two groups: conservative force and non-conservative force. A force is conservative if the total work done by it on a particle moving in a closed path is zero. In other words, the work done by a conservative force on a particle is independent of the path it takes to move from one point to another. Examples: force of gravity, spring force.
The energy diagram is the graph that shows the variation in the potential energy U(x) of a system with the displacement x of a particle forming part of the system. Energy diagram helps us to determine whether a body is in stable equilibrium, unstable equilibrium or neutral equilibrium.
According to the principle of conservation of mechanical energy, the total mechanical energy of an isolated system of particles which interact only through conservative forces is constant. If K1 , U1 are the initial values and K2 , U2 are the final values of the kinetic and potential energies of the system then:
K1 + U1 = K2 + U2
The principle can be applied to solve a host of mechanical problems. The method is often referred to as the energy method of solving a problem. It is usually more elegant and easier than force-based dynamical method of solving a problem.
Another conservation principle with broader scope is the principle of conservation of energy. It states that energy can neither be created nor destroyed. It may be transformed from one form to another, but the total energy of an isolated system is always constant. From a universal point of view, the total energy of the universe is constant.
Any discussion on energy conservation remains incomplete without reference to Einstein's famous formula on equivalence of mass and energy:
E0 = m0 c2
In this expression, m0 is the rest mass of a particle, E0 is the rest energy which is energy equivalent of m0 , and c is the speed of light in vacuum.
As the title suggests, the topic can be broadly divided into three sections. In the first section, we define the impulse of a force and state the impulse-momentum theorem. In the second section, we develop the principle of conservation of linear momentum and apply that to study collision between two bodies. In the third and final section, we define centre of mass of a system of particles and determine its position for various cases.
The impulse of a constant force is given by the product of the force and its duration. If the force is F and its duration is ∆ t , the impulse is given by:
I = F ∆ t
Impulse is a vector; its direction is the same as that of of the force. The dimensions of impulse are [MLT-1], and its SI unit is kilogram metre per second (kg-m/s).
Suppose the momentum of a particle changes from p1 at time t1 to p2 at time t2 under a force F applied over the interval ∆ t = t2 - t1.
It can be shown from Newton's second law of motion that:
I = F ∆ t = p2 - p1 = ∆ p
In words, the impulse of a force acting on a particle is equal to the change in linear momentum of the particle. This is the statement of the impulse-momentum theorem. If the force varies in magnitude and/or direction with time, the deduction becomes a little complicated. However, the impulse-momentum theorem holds. This last result shows that, for a given change in momentum of a particle, the force is inversely proportional to its duration. We should mention impulsive force in this context. Blows, collisions and explosions involve this type of force whose magnitude is very large and duration is extremely small.
Impulse-momentum theorem can be successfully applied to study the motion of a body of variable mass such as a rocket. The fundamental equation of motion of a body of variable mass is:
F = (v - u) dm/dt + m dv/dt
If the motion happens along a straight line, the scalar equivalent of the above equation is:
F = (v - u) dm/dt + m dv/dt
While other symbols have their usual meanings, dm/dt stands for rate of change in mass with time and dv/dt stands for rate of change in velocity with time. Some examples worth studying are a leaking sand-filled wagon on a frictionless track, sand pouring onto a conveyor belt and, of course, motion of a rocket.
Before we state the principle of conservation of linear momentum, a few terms need to be defined. A system is a well-defined collection of particles under investigation. All other particles in the universe except the system compose the surroundings.
Consider two interacting particles 1 and 2. The force exerted by 1 on 2 is F21 and that exerted by 2 on 1 is F12. If the system consists of only 1, 2 forms part of the surroundings and F12 is an external force on the system. Now, let us redefine the system such that it consists of both 1 and 2. In that case, both F21 and F12 have their sources within the system and are called internal forces. A system on which no net external force acts is called an isolated system.
The principle of conservation of linear momentum states that the total linear momentum of an isolated system remains constant. For a two-particle system, the mathematical statement of the principle would be:
m1 u1 + m2 u2 = m1 v1 + m2 v2
The symbols have their usual meanings.
Collision is defined as an event in which two (or more) particles come together for a short time and exert relatively large forces on each other to produce an abrupt change in motion of at least one of them. It usually implies a physical contact between colliding particles such as two billiard balls. However, this is not an essential condition. An alpha particle (He++) directed towards a gold nucleus deviates sharply from its original path even before any physical contact. Yet they are said to have "collided" because of the strong, brief electrostatic force of repulsion between them.
The coefficient of restitution, e, of a collision is a measure of the restitution (tendency to return to original shape) of the involved particles. It is given by the ratio of the velocity of separation after the collision to the velocity of approach before the collision. For a perfectly elastic collision, e = 1. For a perfectly inelastic collision, e = 0. For a partly elastic collision, 0 < e < 1. Most real collisions belong to this last category.
The centre of mass of a system of particles is defined as a point where the whole mass of the system may
be assumed to be concentrated such that the point would move in the same way as a single particle subjected to the
same external force would move.
Let us consider a system of n particles. The masses of the particles are m1, m2, …, mn.
Therefore, the total mass of the system of particles is M = m1 + m2 + … + mn.
At a given instant, the position coordinates of the particles in space are
(x1, y1, z1),
(x2, y2, z2), .... (xn, yn, zn).
At the same instant, the position coordinates of the centre of mass of the system will be:
xcm = (m1x1 + m2x2 + … + mnxn) / M
ycm = (m1y1 + m2y2 + … + mnyn) / M
Zcm = (m1z1 + m2z2 + … + mnzn) / M
For uniform rigid bodies of simple shape, the centre of mass can be located by inspection alone. For example, the centre of mass of a uniform rod coincides with its mid-point. The centre of mass of a uniform disc coincides with its geometric centre. The centre of mass of a uniform triangular plate coincides with its centroid, which is the intersection of the three medians.
For nonuniform rigid bodies or for uniform rigid bodies of not-so-simple shape, the centre of mass can be located by the method of integration. Examples of interest are nonuniform rod, uniform semicircular wire, semicircular plate, solid hemisphere etc, as we shall see in due course.
There are many instances of rotation around us. As a rotating body moves from one position to another, a straight line joining any two points on the body makes an angle with its previous position. This is an important criterion which helps to distinguish between rotation and certain kinds of curvilinear motion. In the first part, we discuss rotation about a fixed axis, otherwise known as pure rotation. The motion of a fan blade, a gramophone record, the steering wheel of a car etc is of this type. The second part deals with rotation about a moving axis which, in effect, is a combination of translation and rotation. A sphere rolling on the floor or a yo-yo bouncing up and down performs this kind of motion.
When a rigid body performs pure rotation, one line in the body (or body extended) remains fixed in space while all points not lying on the line move in circles, each having its centre on the fixed line. We call this line the axis of rotation. Because of this close connection between circular motion and rotational motion, the kinematic equations of circular motion developed earlier are also valid for rotation of a rigid body. As you will recall, the equations involve quantities such as angular displacement, angular velocity and angular acceleration.
The rotational effect of a force depends not only on its magnitude, but also on the position of its line of action. The effect is measured in terms of a physical quantity called torque or moment of a force. Suppose P is the position of a particle in the xy-plane. The line of action of the force F acting on the particle lies in the same plane. The position vector of P with respect to the origin O is r. Let the angle between the vectors r and F be θ. The torque τ of F about O is defined as the cross product of r and F. That is:
τ = r × F
The magnitude of the torque vector is given by:
τ = rF sinθ = Fd
where d = r sinθ is the perpendicular distance from O to the line of action of F.
The quantity d is known as the moment arm of F about O.
The direction of the torque follows from right-hand corkscrew rule. The dimensions of torque are
[ML2T-2] , and its SI unit is newton-metre (N-m).
Two equal and opposite forces having parallel lines of action form a couple. While the resultant of these two forces is zero, the net torque produced by them about any point is never zero.
The equation of rotational motion of a rigid body is:
τext = Iα
In the above expression, τext is the torque associated with external forces acting on the body, I is the moment of inertia of the body about the axis of rotation and α is its angular acceleration.
Recognize that this equation is the rotational analogue of F = ma, applied to the rectilinear motion of a particle.
A detailed study of moment of inertia will take us through the definition of radius of gyration and calculation of moment of inertia of various bodies by the method of integration. Two useful theorems on moment of inertia – parallel axes theorem and perpendicular axes theorem – will also be discussed.
Just like linear momentum is important in the discussion of translational motion, angular momentum
is important in the discussion of rotational motion. Consider a particle of mass m moving in the xy-plane. At some instant,
the particle is at the point P whose position vector with respect to the origin O is r. The velocity of the
particle at this instant is v, and therefore, its linear momentum is p = mv.
Let the angle between the vectors r and p be θ.
The angular momentum, l, of the particle about O is defined as the cross product of r and p. That is:
l = r × p
The magnitude of the angular momentum vector is given by:
l = rp sinθ = mvr sinθ
The direction of l is given, as usual, by the right-hand corkscrew rule.
According to the principle of conservation of angular momentum, the total angular momentum of a system of particles remains constant if the net external torque acting on the system is zero.
So far we discussed rotation of a rigid body about a fixed axis. We may now consider more general cases where the axis of rotation moves in space. Take the case of a wheel rolling on a horizontal straight road. An observer standing on the ground sees that the wheel rotates about a horizontal axis through the centre of mass and, at the same time, the centre of mass itself moves along a straight line. So this becomes a case of combined translational and rotational motion. A few cases worth analysing are rolling motion of a rigid body on horizontal floor and down an incline, a cylinder falling at the end of an unwinding string etc.
A body is said to have attained mechanical equilibrium if it either remains at rest or moves with a constant velocity. The branch of physics which studies the conditions of equilibrium of a body at rest is called statics. Strictly speaking, all bodies which attain equilibrium under a set of forces are deformed to a certain extent. However, for comparatively small forces, we may ignore the small deformations and treat the body under investigation as a perfectly rigid body.
Let us consider a three-dimensional rigid body of arbitrary shape in a frame of reference, Oxyz. A set of n external forces F1, F2, …, Fn are acting on the body. The static equilibrium of the rigid body requires the following conditions to be satisfied separately. The condition of translational equilibrium is:
∑ F = F1 + F2 + … + Fn = 0
If τ1, τ2, …, τn are the individual torques produced by the above forces about the origin O, the condition of rotational equilibrium is:
∑ τ = τ1 + τ2 + … + τn = 0
The torque equation is valid for any choice of origin inside or outside the boundaries of the body, provided the force equation holds good.
The number of velocity components required to completely describe the motion of a body is called the number of degrees of freedom of the body. An insect has one degree of freedom while walking along a straight wire, two degrees of freedom while crawling all over a flat table top, and a maximum of three degrees of freedom while flying randomly in the air. Treating the insect as a particle, all these degrees of freedom are associated with translational motion only. An extended body on the other hand is capable of both translation and rotation. Each type of motion contributes one to three degrees of freedom. In the most general case, an extended body acted upon by a set of non-coplanar forces has six degrees of freedom – three translational and three rotational. Now, each degree of freedom corresponds to one independent condition of equilibrium. In other words, the number of degrees of freedom is equal to the number of conditions of static equilibrium of a rigid body. Our video lectures mostly deal with simple cases involving coplanar forces and few equations, such as the equilibrium of a leaning ladder.
A statically indeterminate problem is one in which the number of unknown quantities is higher than the number of independent equations of equilibrium involving those unknowns. Obviously, such a problem cannot be solved. This type of situation arises when a body gets one or more extra support(s) over and above the minimum required for its equilibrium. An interesting example is a ladder resting on a rough floor against a rough wall.
The centre of gravity of a body is a fixed point through which the resultant of the forces of gravity acting on the constituent particles of the body passes, regardless of how the body is oriented in space. The centre of gravity and the centre of mass of a body are located at the same point, provided the body is placed in a uniform gravitational field. For exceptionally large bodies, such as a mountain, these two points do not coincide.
The stability of static equilibrium of a body can be determined as follows. Let the body be slightly tilted from its position of equilibrium. If a restoring torque comes into play and brings the body back to its initial position, the equilibrium is stable. If a destabilising torque comes into play and pushes the body further from its initial position, the equilibrium is unstable.
Newton's Law of gravitation states that every particle in the universe attracts every other particle with a force which is directly proportional to the mass of each particle and inversely proportional to the square of the distance between them. If two particles 1 and 2 have masses m1 and m2, and, the distance between them is r, the magnitude of the gravitational force between them is:
Fg = Gm1m2 /r2
The constant of proportionality G is called universal gravitational constant, and its value is 6.67 × 10-11 N-m2/ kg2.
Force of gravity is the special name given to the gravitational force exerted by the earth on any body on or near its surface. This force produces an acceleration due to gravity, g, in the body. The vector g is always directed towards the centre of the earth and its standard value at sea level at 45° latitude is 9.81 m/s2 . Variation of acceleration due to gravity happens due to a number of factors such as altitude, depth, earth's rotation and its non-spherical shape.
A body possesses two types of mass: inertial mass and gravitational mass. The concept of inertial mass comes from Newton's second law of motion. It gives a measure of resistance to any change of state of rest or motion under an external force. The concept of gravitational mass arises from Newton's Law of gravitation. It gives a measure of the force experienced by the body in a given gravitational field. Experiment shows inertial mass and gravitational mass of a body are the same.
Kepler's laws of planetary motion, as published by Johannes Kepler between 1609 and 1619, are as follows:
The gravitational field at a point in space is the gravitational force experienced by a unit mass placed at that point. The dimensions of gravitational field are [LT-2], and its SI unit is newton per kilogram (N/kg).
The gravitational potential energy of a system comprising two particles of masses m1 and m2 separated by distance r is:
U = - G m1m2 / r
This is a negative quantity, which becomes zero if the particles are at infinite separation.
The gravitational potential at a point due to a particle is the gravitational potential energy of a system comprising the particle in question and a unit mass placed at the given point. The dimensions of gravitational potential are [L2T-2], and its SI unit is joule per kilogram (J/kg).
The minimum speed with which a particle must be projected so that it may escape the gravitational attraction of the earth is called the escape speed for the earth. It is given by the formula:
vesc = √(2GME /RE)
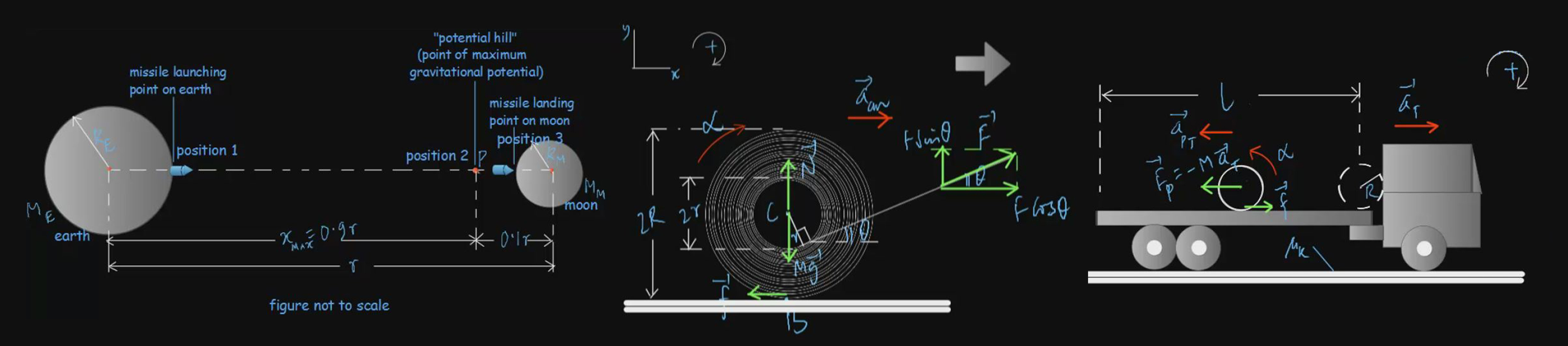
where ME and RE are the mass and radius of the earth respectively. Its value is 11.2 km/s, independent of the angle of projection of the particle. Other heavenly bodies such as the sun, other planets and their moons also have their respective escape speeds. Escape speed assigned with a specific direction is called escape velocity.
Just like the planets revolve round the sun, many of the planets have one or more natural satellites revolving round them. A natural satellite is also called a "moon". The earth's moon is its only natural satellite. However, man has launched many artificial satellites in orbits round the earth. The orbital speed of a satellite in a circular orbit round the earth is given by:
vorb = √(GME / r)
Here r = RE + h is the orbit radius, h being the height of orbit above earth's surface. Orbital velocity at a given point on the orbit has magnitude equal to orbital speed and direction along the tangent to the orbit at the given point.
A geostationary satellite is an artificial satellite which always remains above the same spot on the equator. Its orbit is called geosynchronous orbit or parking orbit.
The total mechanical energy of satellite-earth system is given by:
E = - GME m / 2r
where ME is earth's mass, m is satellite's mass, and r is orbit radius.
Since a satellite revolving round the earth is in a state of free fall, the satellite and its occupants must also be weightless. An astronaut, like any other human, feels comfortable with the sensation of weight. Therefore, weightlessness of an astronaut during their long stay in an orbiting satellite is an unpleasant feeling and may cause medical symptoms.
We are confident of getting your support and appreciation. That will inspire us to add new features to our website, such as question & problem banks, doubt removal sessions etc. Please let me know your feedback
Hearty welcome to Physicsacademyonline.in / PhysicsAcademyOnline.com /PhysicsAcademyOnline.org / PhysicsAcademyOnline.info / PhysicsAcademyOnline.net.